Talk:Modular equivalence
Mod notation can be bulky and counter-intuitive. Here is an alternate mod notation that simply removes the middle line from the equivalence sign, places the modulus inside and drops the now moot 'mod'.
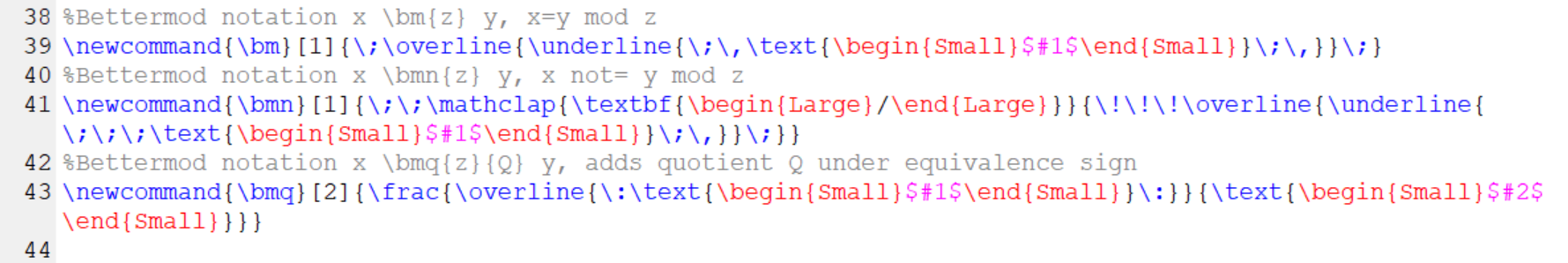
The following macros are defined for the Latex that follows and the second graphic shows the result:
Examples: In lieu of $x\equiv y \mod{z}$, we have $x\bm{z}y$ Rather than take both sides $\mod{m}$, we would take both sides $\bm{m}$ Taking all $m\bm{7}1\in \mathbb{Z}_{+}$ gives $\{1,8,15,\dots\}$ A non-equivalence can be written $9\bmn{4}3$ It can be chained ala $27\bm{11}5\bm{3}2$ and even be adapted to carry quotient information as in $77\bmq{13}{5}12$.
Here's how that looks when rendered:
This notation is more compact, intuitive, flexible, prettier, puts the modulus in the middle where it belongs and there is no dead mathematician named Mod being dishonored and forgotten and even if there were, he or she should be.